Figure 8.1

The area under the curve.

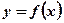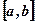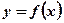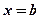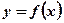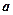Suppose is nonnegative, continuous throughout. We want to define the area of the region enclosed by the graph of the function, the -axis and the vertical lines and . We call this area the area under the curve from to .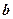

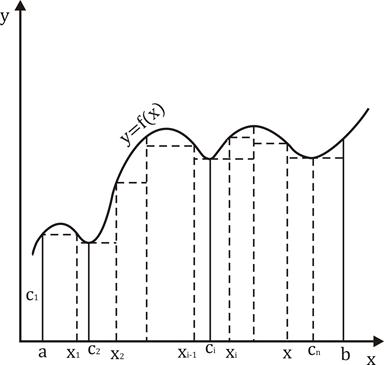
We partition 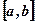into 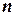subintervals of the length by choosing the points 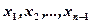such that

.

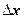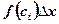The vertical lines through the points divide the region into vertical strips. We approximate each strip with an inscribed rectangle with base and the height which is equal to the minimum value of the function on the rectangle base. So, the area of -th rectangle equals and the sum of the areas of the rectangles will be

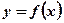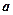This formula approximates the area under the curve from to .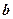

The area approximation given by formulamay not be particularly good if the number of the rectangles is small. To increase approximation we can by approaching the number 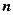to the infinity

Дата добавления: 2014-01-11